Aufgaben:Aufgabe 1.3: Rechnen mit komplexen Zahlen: Unterschied zwischen den Versionen
Aus LNTwww
| (3 dazwischenliegende Versionen desselben Benutzers werden nicht angezeigt) | |||
| Zeile 4: | Zeile 4: | ||
Nebenstehende Grafik zeigt einige Punkte in der komplexen Ebene, nämlich | Nebenstehende Grafik zeigt einige Punkte in der komplexen Ebene, nämlich | ||
| − | :$$z_1 = {\rm e}^{\hspace{0.05cm}-{\rm j} 45^{ \circ}}, $$ | + | :$$z_1 = {\rm e}^{\hspace{0.05cm}-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}}, $$ |
| − | :$$z_2 = 2 \cdot{\rm e}^{\hspace{0.05cm}{\rm j} 135^{ \circ}},$$ | + | :$$z_2 = 2 \cdot{\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}},$$ |
:$$z_3 = -{\rm j} .$$ | :$$z_3 = -{\rm j} .$$ | ||
| Zeile 15: | Zeile 15: | ||
:$$z_8 = {\rm e}^{\hspace{0.05cm}z_2} + {\rm e}^{\hspace{0.05cm}z_2^{\star}}.$$ | :$$z_8 = {\rm e}^{\hspace{0.05cm}z_2} + {\rm e}^{\hspace{0.05cm}z_2^{\star}}.$$ | ||
| − | |||
''Hinweise:'' | ''Hinweise:'' | ||
| − | *Die Aufgabe gehört zum Kapitel [[Signaldarstellung/Zum_Rechnen_mit_komplexen_Zahlen|Zum Rechnen mit komplexen Zahlen]]. | + | *Die Aufgabe gehört zum Kapitel [[Signaldarstellung/Zum_Rechnen_mit_komplexen_Zahlen|Zum Rechnen mit komplexen Zahlen]]. |
| − | *Die Thematik wird auch im Lernvideo [[Rechnen_mit_komplexen_Zahlen_(Lernvideo)|Rechnen mit komplexen Zahlen ]] behandelt. | + | *Die Thematik wird auch im Lernvideo [[Rechnen_mit_komplexen_Zahlen_(Lernvideo)|Rechnen mit komplexen Zahlen ]] behandelt. |
| Zeile 27: | Zeile 26: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {Welche der folgenden Gleichungen sind | + | {Welche der folgenden Gleichungen sind richtig? |
|type="[]"} | |type="[]"} | ||
+ <math>2 \cdot z_1 + z_2 =0.</math> | + <math>2 \cdot z_1 + z_2 =0.</math> | ||
| Zeile 34: | Zeile 33: | ||
| − | {Welchen Wert besitzt die Zufallsgröße <math>z_4 = z_2^2 + z_3^2 = x_4 + {\rm j} \cdot y_4</math>? | + | {Welchen Wert besitzt die Zufallsgröße <math>z_4 = z_2^2 + z_3^2 = x_4 + {\rm j} \cdot y_4</math>? |
|type="{}"} | |type="{}"} | ||
<math> x_4 \ =\ </math> { -1.01--0.99 } | <math> x_4 \ =\ </math> { -1.01--0.99 } | ||
| Zeile 40: | Zeile 39: | ||
| − | {Berechnen Sie die komplexe Größe <math>z_5 = 1/z_2 = x_5 + {\rm j} \cdot y_5</math>. | + | {Berechnen Sie die komplexe Größe <math>z_5 = 1/z_2 = x_5 + {\rm j} \cdot y_5</math>. |
|type="{}"} | |type="{}"} | ||
<math> x_5 \ =\ </math> { -0.36--0.35 } | <math> x_5 \ =\ </math> { -0.36--0.35 } | ||
<math> y_5 \ =\ </math> { -0.36--0.35 } | <math> y_5 \ =\ </math> { -0.36--0.35 } | ||
| − | {<math>z_6</math> hat als Quadratwurzel von <math>z_3</math> zwei Lösungen, beide mit dem Betrag <math>|z_6| = 1</math>. Geben Sie die beiden möglichen Phasenwinkel von <math>z_6</math> an. | + | {<math>z_6</math> hat als Quadratwurzel von <math>z_3</math> zwei Lösungen, beide mit dem Betrag <math>|z_6| = 1</math>. <br>Geben Sie die beiden möglichen Phasenwinkel von <math>z_6</math> an. |
|type="{}"} | |type="{}"} | ||
| − | <math> \phi_6 ({\rm zwischen\hspace{0.1cm} 0^{\circ} \hspace{0.1cm}und \hspace{0.1cm} +\hspace{-0.15cm}180^{\circ} \hspace{0.1cm}Grad}) \hspace{0.2cm} =\ </math> { 133-137 } $\text{Grad}$ | + | <math> \phi_6 \ ({\rm zwischen\hspace{0.1cm} 0^{\circ} \hspace{0.1cm}und \hspace{0.1cm} +\hspace{-0.15cm}180^{\circ} \hspace{0.1cm}Grad}) \hspace{0.2cm} =\ </math> { 133-137 } $\ \text{Grad}$ |
| − | <math> \phi_6 ({\rm zwischen\hspace{0.1cm} - \hspace{-0.15cm}180^{\circ} \hspace{0.1cm}und \hspace{0.1cm} 0^{\circ} \hspace{0.1cm}Grad}) \hspace{0.2cm} =\ </math> { -47--43 } $\text{Grad}$ | + | <math> \phi_6 \ ({\rm zwischen\hspace{0.1cm} - \hspace{-0.15cm}180^{\circ} \hspace{0.1cm}und \hspace{0.1cm} 0^{\circ} \hspace{0.1cm}Grad}) \hspace{0.2cm} =\ </math> { -47--43 } $\ \text{Grad}$ |
| − | {Berechnen Sie <math>z_7 = {\rm e}^{z_2} = x_7 + {\rm j} \cdot y_7</math>. | + | {Berechnen Sie <math>z_7 = {\rm e}^{z_2} = x_7 + {\rm j} \cdot y_7</math>. |
|type="{}"} | |type="{}"} | ||
<math> x_7 \ =\ </math> { 0.03-0.04 } | <math> x_7 \ =\ </math> { 0.03-0.04 } | ||
| Zeile 57: | Zeile 56: | ||
| − | {Geben Sie die komplexe Größe <math>z_8 = {\rm e}^{z_2} + {\rm e}^{z_2^{\ast}} = x_8 + {\rm j}\cdot y_8</math> an. | + | {Geben Sie die komplexe Größe <math>z_8 = {\rm e}^{z_2} + {\rm e}^{z_2^{\ast}} = x_8 + {\rm j}\cdot y_8</math> an. |
|type="{}"} | |type="{}"} | ||
<math> x_8 \ =\ </math> { 0.07-0.08 } | <math> x_8 \ =\ </math> { 0.07-0.08 } | ||
| Zeile 67: | Zeile 66: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' Richtig sind | + | '''(1)''' Richtig sind die<u> Lösungsvorschläge 1 und 2</u>: |
| − | *Entsprechend den Angaben gilt mit dem [[Signaldarstellung/Zum_Rechnen_mit_komplexen_Zahlen#Darstellung_nach_Betrag_und_Phase|Satz von Euler]]: | + | *Entsprechend den Angaben gilt mit dem [[Signaldarstellung/Zum_Rechnen_mit_komplexen_Zahlen#Darstellung_nach_Betrag_und_Phase|Satz von Euler]]: |
| − | ::<math>2 \cdot z_1 + z_2 = 2 \cdot \cos(45^{ \circ}) - 2{\rm j}\cdot \sin(45^{ \circ})- 2 \cdot \cos(45^{ \circ}) + 2{\rm j} \cdot\sin(45^{ \circ}) = 0.</math> | + | ::<math>2 \cdot z_1 + z_2 = 2 \cdot \cos(45^{ \circ}) - 2 \cdot {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} \sin(45^{ \circ})- 2 \cdot \cos(45^{ \circ}) + 2\cdot {\rm j} \cdot\sin(45^{ \circ}) = 0.</math> |
| − | Der zweite Vorschlag ist ebenfalls richtig, da | + | *Der zweite Vorschlag ist ebenfalls richtig, da |
| − | ::<math>z_1^{\star} \cdot z_2 = 1 \cdot{\rm e}^{{\rm j} 45^{ \circ}} \cdot 2 \cdot{\rm e}^{{\rm j} 135^{ \circ}} = 2 \cdot{\rm e}^{{\rm j} 180^{ | + | ::<math>z_1^{\star} \cdot z_2 = 1 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}} \cdot 2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ \circ}} = 2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 180^{ |
\circ}}= -2.</math> | \circ}}= -2.</math> | ||
| − | Dagegen ist der dritte Vorschlag falsch. Die Division von <math>z_1</math> und <math>z_2</math> liefert: | + | *Dagegen ist der dritte Vorschlag falsch. Die Division von <math>z_1</math> und <math>z_2</math> liefert: |
| − | ::<math>\frac{z_1}{z_2} = \frac{{\rm e}^{-{\rm j} 45^{ \circ}}}{2 \cdot{\rm e}^{{\rm j} 135^{ \circ}}} = | + | ::<math>\frac{z_1}{z_2} = \frac{{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}}}{2 \cdot{\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}}} = |
| − | 0.5 \cdot{\rm e}^{-{\rm j} 180^{ | + | 0.5 \cdot{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 180^{ |
\circ}}= -0.5.</math> | \circ}}= -0.5.</math> | ||
| − | Die Multiplikation mit <math>z_3 = -{\rm j} </math> führt zum Ergebnis j/2, also zu einer rein imaginären Größe. | + | *Die Multiplikation mit <math>z_3 = -{\rm j} </math> führt zum Ergebnis ${\rm j}/2$, also zu einer rein imaginären Größe. |
| + | |||
| − | '''(2)''' Das Quadrat von <math>z_2</math> hat den Betrag <math>|z_2|^{2}</math> und die Phase <math>2 \cdot \phi_2</math>: | + | '''(2)''' Das Quadrat von <math>z_2</math> hat den Betrag <math>|z_2|^{2}</math> und die Phase <math>2 \cdot \phi_2</math>: |
| − | ::<math>z_2^2 = 2^2 \cdot{\rm e}^{{\rm j} 270^{ \circ}}= 4 \cdot {\rm e}^{-{\rm j} 90^{ \circ}}=-4 \cdot {\rm j}.</math> | + | ::<math>z_2^2 = 2^2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 270^{ \circ}}= 4 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 90^{ \circ}}=-4 \cdot {\rm j}.</math> |
| − | Entsprechend gilt für das Quadrat von <math>z_3</math>: | + | *Entsprechend gilt für das Quadrat von <math>z_3</math>: |
::<math>z_3^2 = (-{\rm j})^2 = -1.</math> | ::<math>z_3^2 = (-{\rm j})^2 = -1.</math> | ||
| − | Somit ist <math>x_4 =\underline{ –1}</math> und <math>y_4 = \underline{–4}.</math> | + | *Somit ist <math>x_4 =\underline{ –1}</math> und <math>y_4 = \underline{–4}.</math> |
| + | |||
'''(3)''' Durch Anwendung der Divisionsregel erhält man: | '''(3)''' Durch Anwendung der Divisionsregel erhält man: | ||
| − | ::<math>z_5 = {1}/{z_2} = \frac{1}{2 \cdot{\rm e}^{{\rm j} 135^{ \circ}}}= 0.5 \cdot{\rm e}^{-{\rm j} 135^{ | + | ::<math>z_5 = {1}/{z_2} = \frac{1}{2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ \circ}}}= 0.5 \cdot{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ |
| − | \circ}} = 0.5 \cdot \ | + | \circ}} = 0.5 \cdot \big[ \cos (- 135^{ \circ}) + {\rm j} \cdot \sin (- 135^{ |
| − | \circ})\ | + | \circ})\big]</math> |
| − | ::<math>\Rightarrow x_5 = - {\sqrt{2}}/{4}\underline{= -0.354},\hspace{0.5cm} y_5 = x_5 \underline{= -0.354}.</math> | + | ::<math>\Rightarrow \ x_5 = - {\sqrt{2}}/{4}\hspace{0.15cm}\underline{= -0.354},\hspace{0.5cm} y_5 = x_5 \hspace{0.15cm}\underline{= -0.354}.</math> |
| − | + | '''(4)''' Die angegeben Beziehung für <math>z_6</math> kann wie folgt umgeformt werden: <math>z_6^2 = {z_3} = {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 90^{ \circ}}.</math> | |
| − | Man erkennt, dass es zwei Möglichkeiten für <math>z_6</math> gibt, die diese Gleichung erfüllen: | + | *Man erkennt, dass es zwei Möglichkeiten für <math>z_6</math> gibt, die diese Gleichung erfüllen: |
| − | ::<math>z_6 \hspace{0.1cm}{\rm (1.\hspace{0.1cm} L\ddot{o}sung)}\hspace{0.1cm} = \frac{z_2}{2} = 1 \cdot {\rm e}^{{\rm j} 135^{ \circ}} | + | ::<math>z_6 \hspace{0.1cm}{\rm (1.\hspace{0.1cm} L\ddot{o}sung)}\hspace{0.1cm} = \frac{z_2}{2} = 1 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}} |
\hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{= 135^{ | \hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{= 135^{ | ||
\circ}}, </math> | \circ}}, </math> | ||
| − | ::<math>z_6 \hspace{0.1cm}{\rm (2.\hspace{0.1cm} L \ddot{o}sung)}\hspace{0.1cm} = {z_1} = 1 \cdot {\rm e}^{-{\rm j} 45^{ \circ}} | + | ::<math>z_6 \hspace{0.1cm}{\rm (2.\hspace{0.1cm} L \ddot{o}sung)}\hspace{0.1cm} = {z_1} = 1 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}45^{ \circ}} |
\hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{=-45^{ | \hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{=-45^{ | ||
\circ}}.</math> | \circ}}.</math> | ||
| − | '''(5)''' Die komplexe Größe <math>z_2</math> lautet in Realteil/ | + | '''(5)''' Die komplexe Größe <math>z_2</math> lautet in Realteil/Imaginärteildarstellung: |
::<math>z_2 = x_2 + {\rm j} \cdot y_2 = -\sqrt{2} + {\rm j} \cdot\sqrt{2}.</math> | ::<math>z_2 = x_2 + {\rm j} \cdot y_2 = -\sqrt{2} + {\rm j} \cdot\sqrt{2}.</math> | ||
| − | Damit ergibt sich für die komplexe Exponentialfunktion: | + | *Damit ergibt sich für die komplexe Exponentialfunktion: |
| − | ::<math>z_7 = {\rm e}^{-\sqrt{2} + {\rm j}\cdot \sqrt{2}}= {\rm e}^{-\sqrt{2} } \cdot \ | + | ::<math>z_7 = {\rm e}^{-\sqrt{2} + {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}\sqrt{2}}= {\rm e}^{-\sqrt{2} } \cdot \big[ \cos (\sqrt{2}) + {\rm j} \cdot \sin (\sqrt{2})\big].</math> |
| − | Mit <math>{\rm e}^{-\sqrt{2} } = 0.243, \hspace{0. | + | *Mit <math>{\rm e}^{-\sqrt{2} } = 0.243, \hspace{0.4cm} \cos (\sqrt{2}) = 0.156, \hspace{0.4cm} \sin (\sqrt{2}) = 0.988</math> erhält man somit: |
::<math>z_7 = 0.243 \cdot \left( 0.156 + {\rm j} \cdot 0.988\right) \hspace{0.15cm}\underline{= 0.038 + {\rm j} \cdot 0.24}.</math> | ::<math>z_7 = 0.243 \cdot \left( 0.156 + {\rm j} \cdot 0.988\right) \hspace{0.15cm}\underline{= 0.038 + {\rm j} \cdot 0.24}.</math> | ||
| − | |||
| − | ::<math>z_8 = {\rm e}^{-\sqrt{2} } \cdot \ | + | '''(6)''' Ausgehend vom Ergebnis der Teilaufgabe '''(4)''' erhält man für <math>z_8</math>: |
| − | (\sqrt{2})\ | + | |
| − | = 2 \cdot {\rm e}^{-\sqrt{2} } \cdot \cos (\sqrt{2}) = 2 \cdot x_7 \hspace{0. | + | ::<math>z_8 = {\rm e}^{-\sqrt{2} } \cdot \big[ \cos (\sqrt{2}) + {\rm j} \cdot \sin (\sqrt{2}) + \cos (\sqrt{2}) - {\rm j} \cdot \sin |
| + | (\sqrt{2})\big] | ||
| + | = 2 \cdot {\rm e}^{-\sqrt{2} } \cdot \cos (\sqrt{2}) = 2 \cdot x_7 \hspace{0.5cm}\Rightarrow \hspace{0.5cm} x_8 \hspace{0.15cm}\underline{= 0.076}, \hspace{0.4cm}y_8\hspace{0.15cm}\underline{ = 0}.</math> | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
[[Category:Aufgaben zu Signaldarstellung|^1. Grundbegriffe der Nachrichtentechnik^]] | [[Category:Aufgaben zu Signaldarstellung|^1. Grundbegriffe der Nachrichtentechnik^]] | ||
Aktuelle Version vom 9. April 2021, 15:39 Uhr
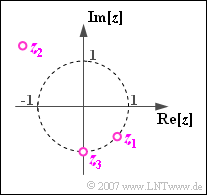
Nebenstehende Grafik zeigt einige Punkte in der komplexen Ebene, nämlich
- $$z_1 = {\rm e}^{\hspace{0.05cm}-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}}, $$
- $$z_2 = 2 \cdot{\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}},$$
- $$z_3 = -{\rm j} .$$
Im Verlauf dieser Aufgabe werden noch folgende komplexe Größen betrachtet:
- $$z_4 = z_2^2 + z_3^2,$$
- $$z_5 = 1/z_2,$$
- $$z_6 = \sqrt{z_3},$$
- $$z_7 = {\rm e}^{\hspace{0.05cm}z_2},$$
- $$z_8 = {\rm e}^{\hspace{0.05cm}z_2} + {\rm e}^{\hspace{0.05cm}z_2^{\star}}.$$
Hinweise:
- Die Aufgabe gehört zum Kapitel Zum Rechnen mit komplexen Zahlen.
- Die Thematik wird auch im Lernvideo Rechnen mit komplexen Zahlen behandelt.
Fragebogen
Musterlösung
(1) Richtig sind die Lösungsvorschläge 1 und 2:
- Entsprechend den Angaben gilt mit dem Satz von Euler:
- \[2 \cdot z_1 + z_2 = 2 \cdot \cos(45^{ \circ}) - 2 \cdot {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} \sin(45^{ \circ})- 2 \cdot \cos(45^{ \circ}) + 2\cdot {\rm j} \cdot\sin(45^{ \circ}) = 0.\]
- Der zweite Vorschlag ist ebenfalls richtig, da
- \[z_1^{\star} \cdot z_2 = 1 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}} \cdot 2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ \circ}} = 2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 180^{ \circ}}= -2.\]
- Dagegen ist der dritte Vorschlag falsch. Die Division von \(z_1\) und \(z_2\) liefert:
- \[\frac{z_1}{z_2} = \frac{{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 45^{ \circ}}}{2 \cdot{\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}}} = 0.5 \cdot{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 180^{ \circ}}= -0.5.\]
- Die Multiplikation mit \(z_3 = -{\rm j} \) führt zum Ergebnis ${\rm j}/2$, also zu einer rein imaginären Größe.
(2) Das Quadrat von \(z_2\) hat den Betrag \(|z_2|^{2}\) und die Phase \(2 \cdot \phi_2\):
- \[z_2^2 = 2^2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 270^{ \circ}}= 4 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 90^{ \circ}}=-4 \cdot {\rm j}.\]
- Entsprechend gilt für das Quadrat von \(z_3\):
- \[z_3^2 = (-{\rm j})^2 = -1.\]
- Somit ist \(x_4 =\underline{ –1}\) und \(y_4 = \underline{–4}.\)
(3) Durch Anwendung der Divisionsregel erhält man:
- \[z_5 = {1}/{z_2} = \frac{1}{2 \cdot{\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ \circ}}}= 0.5 \cdot{\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 135^{ \circ}} = 0.5 \cdot \big[ \cos (- 135^{ \circ}) + {\rm j} \cdot \sin (- 135^{ \circ})\big]\]
- \[\Rightarrow \ x_5 = - {\sqrt{2}}/{4}\hspace{0.15cm}\underline{= -0.354},\hspace{0.5cm} y_5 = x_5 \hspace{0.15cm}\underline{= -0.354}.\]
(4) Die angegeben Beziehung für \(z_6\) kann wie folgt umgeformt werden: \(z_6^2 = {z_3} = {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} 90^{ \circ}}.\)
- Man erkennt, dass es zwei Möglichkeiten für \(z_6\) gibt, die diese Gleichung erfüllen:
- \[z_6 \hspace{0.1cm}{\rm (1.\hspace{0.1cm} L\ddot{o}sung)}\hspace{0.1cm} = \frac{z_2}{2} = 1 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}135^{ \circ}} \hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{= 135^{ \circ}}, \]
- \[z_6 \hspace{0.1cm}{\rm (2.\hspace{0.1cm} L \ddot{o}sung)}\hspace{0.1cm} = {z_1} = 1 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}45^{ \circ}} \hspace{0.2cm}\Rightarrow \hspace{0.2cm} \phi_6 \hspace{0.15cm}\underline{=-45^{ \circ}}.\]
(5) Die komplexe Größe \(z_2\) lautet in Realteil/Imaginärteildarstellung:
- \[z_2 = x_2 + {\rm j} \cdot y_2 = -\sqrt{2} + {\rm j} \cdot\sqrt{2}.\]
- Damit ergibt sich für die komplexe Exponentialfunktion:
- \[z_7 = {\rm e}^{-\sqrt{2} + {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}\sqrt{2}}= {\rm e}^{-\sqrt{2} } \cdot \big[ \cos (\sqrt{2}) + {\rm j} \cdot \sin (\sqrt{2})\big].\]
- Mit \({\rm e}^{-\sqrt{2} } = 0.243, \hspace{0.4cm} \cos (\sqrt{2}) = 0.156, \hspace{0.4cm} \sin (\sqrt{2}) = 0.988\) erhält man somit:
- \[z_7 = 0.243 \cdot \left( 0.156 + {\rm j} \cdot 0.988\right) \hspace{0.15cm}\underline{= 0.038 + {\rm j} \cdot 0.24}.\]
(6) Ausgehend vom Ergebnis der Teilaufgabe (4) erhält man für \(z_8\):
- \[z_8 = {\rm e}^{-\sqrt{2} } \cdot \big[ \cos (\sqrt{2}) + {\rm j} \cdot \sin (\sqrt{2}) + \cos (\sqrt{2}) - {\rm j} \cdot \sin (\sqrt{2})\big] = 2 \cdot {\rm e}^{-\sqrt{2} } \cdot \cos (\sqrt{2}) = 2 \cdot x_7 \hspace{0.5cm}\Rightarrow \hspace{0.5cm} x_8 \hspace{0.15cm}\underline{= 0.076}, \hspace{0.4cm}y_8\hspace{0.15cm}\underline{ = 0}.\]