Kanalcodierung/Reed–Solomon–Decodierung beim Auslöschungskanal: Unterschied zwischen den Versionen
Ayush (Diskussion | Beiträge) |
|||
| (14 dazwischenliegende Versionen von 3 Benutzern werden nicht angezeigt) | |||
| Zeile 6: | Zeile 6: | ||
}} | }} | ||
| − | == Blockschaltbild und Voraussetzungen | + | == Blockschaltbild und Voraussetzungen zur Reed–Solomon–Fehlererkennung == |
<br> | <br> | ||
| − | Im [ | + | Im Kapitel [[Kanalcodierung/Decodierung_linearer_Blockcodes#Decodierung_beim_Binary_Erasure_Channel|"Decodierung beim Binary Erasure Channel"]] wurde für die binären Blockcodes gezeigt, welche Berechnungen der Decoder ausführen muss, um aus einem unvollständigen Empfangswort $\underline{y}$ das gesendete Codewort $\underline{x}$ bestmöglich decodieren zu können. Im Reed–Solomon–Kapitel haben wir $\underline{x}$ in $\underline{c}$ umbenannt. |
| − | + | [[Datei:P ID2544 KC T 2 4 S1 v2.png|right|frame|Übertragungssystem mit Reed–Solomon–Codierung/Decodierung und Auslöschungskanal|class=fit]] | |
| − | [[ | + | # Zugrunde gelegt wird hier wieder das [[Kanalcodierung/Klassifizierung_von_Signalen#Binary_Erasure_Channel_.E2.80.93_BEC| "BEC–Kanalmodell"]] ("Binary Erasure Channel"), das ein unsicheres Bit als "Erasure" $\rm E$ ("Auslöschung") markiert. |
| + | # Im Gegensatz zu [[Kanalcodierung/Klassifizierung_von_Signalen#Binary_Symmetric_Channel_.E2.80.93_BSC| "BSC-Modell"]] ("Binary Symmetric Channel") und [[Kanalcodierung/Klassifizierung_von_Signalen#AWGN.E2.80.93Kanal_bei_bin.C3.A4rem_Eingang| "AWGN-Modell"]] ("Additive White Gaussian Noise") sind hier Bitfehler $(y_i ≠ c_i)$ ausgeschlossen. | ||
| − | Die Grafik zeigt das Blockschaltbild, das sich von dem Modell | + | |
| − | *Da Reed–Solomon–Codes lineare Blockcodes sind, stehen Informationswort | + | Jedes Bit eines Empfangswortes $\underline{y}$ |
| + | *stimmt also mit dem entsprechenden Bit des Codewortes überein $(y_i = c_i)$, oder | ||
| + | |||
| + | *ist bereits als Auslöschung markiert $(y_i = \rm E)$.<br> | ||
| + | |||
| + | |||
| + | Die Grafik zeigt das Blockschaltbild, das sich von dem Modell im Kapitel [[Kanalcodierung/Decodierung_linearer_Blockcodes#Blockschaltbild_und_Voraussetzungen|"Decodierung linearer Blockcodes"]] geringfügig unterscheidet: | ||
| + | *Da Reed–Solomon–Codes lineare Blockcodes sind, stehen auch hier Informationswort $\underline{u}$ und Codewort $\underline{c}$ über die Generatormatrix $\boldsymbol{\rm G}$ und die folgende Gleichung in Zusammenhang: | ||
::<math>\underline {c} = {\rm enc}(\underline {u}) = \underline {u} \cdot { \boldsymbol{\rm G}} | ::<math>\underline {c} = {\rm enc}(\underline {u}) = \underline {u} \cdot { \boldsymbol{\rm G}} | ||
| − | \hspace{0. | + | \hspace{0.6cm} {\rm mit} \hspace{0.6cm}\underline {u} = (u_0, u_1,\hspace{0.05cm}\text{ ... }\hspace{0.1cm}, u_i, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, u_{k-1})\hspace{0.05cm}, \hspace{0.2cm} |
| − | \underline {c} = (c_0, c_1, ... \hspace{0. | + | \underline {c} = (c_0, c_1, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, c_i, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, c_{n-1}) |
\hspace{0.05cm}.</math> | \hspace{0.05cm}.</math> | ||
| − | *Für die einzelnen Symbole von | + | *Für die einzelnen Symbole von Informationsblock und Codewort gilt bei Reed–Solomon–Codierung: |
| − | ::<math>u_i \in {\rm GF}(q)\hspace{0.05cm},\hspace{0.2cm}c_i \in {\rm GF}(q)\hspace{0. | + | ::<math>u_i \in {\rm GF}(q)\hspace{0.05cm},\hspace{0.2cm}c_i \in {\rm GF}(q)\hspace{0.6cm}{\rm mit}\hspace{0.6cm} q = n+1 = 2^m |
\hspace{0.3cm} \Rightarrow \hspace{0.3cm} n = 2^m - 1\hspace{0.05cm}. </math> | \hspace{0.3cm} \Rightarrow \hspace{0.3cm} n = 2^m - 1\hspace{0.05cm}. </math> | ||
| − | + | *Jedes Codesymbol $c_i$ wird somit mit $m ≥ 2$ Binärsymbolen ("Bit") dargestellt. Zum Vergleich: Für die binären Blockcodes gilt $q=2$, $m=1$ und die Codewortlänge $n$ ist frei wählbar.<br> | |
| − | *Bei Codierung auf Symbolebene muss das BEC–Modell zum | + | *Bei Codierung auf Symbolebene muss das "BEC–Modell" zum "$m$–BEC–Modell" erweitert werden. |
| + | :*Mit der Wahrscheinlichkeit $\lambda_m ≈ m \cdot\lambda$ wird ein Codesymbol $c_i$ ausgelöscht $(y_i = \rm E)$ und es gilt ${\rm Pr}(y_i = c_i) = 1 - \lambda_m$. | ||
| − | + | :*Näheres zur Umrechnung der beiden Modelle finden Sie in der [[Aufgaben:Aufgabe_2.11Z:_Erasure–Kanal_für_Symbole|Aufgabe 2.11Z]].<br> | |
| − | |||
| − | |||
| − | + | Im Folgenden beschäftigen wir uns nur mit dem Block "Codewortfinder" $\rm (CWF)$, der aus dem Empfangsvektor $\underline{y}$ den Vektor $\underline{z} ∈ \mathcal{C}_{\rm RS}$ gewinnt: | |
| + | *Falls die Anzahl $e$ der Auslöschungen im Vektor $\underline{y}$ hinreichend klein ist, lässt sich das gesamte Codewort mit Sicherheit $(\underline{z}=\underline{c})$ finden.<br> | ||
| − | :<math>\underline {c} = \underline {u} \cdot { \boldsymbol{\rm G}} | + | *Sind zuviele Symbole des Empfangswortes $\underline{y}$ ausgelöscht, meldet der Decoder, dass dieses Wort nicht decodierbar ist. Eventuell muss dann diese Sequenz noch einmal gesendet werden.<br><br> |
| + | |||
| + | Beim Auslöschungskanal ($m$–BEC) ist im Gegensatz zum $m$–BSC, der im Kapitel [[Kanalcodierung/Fehlerkorrektur_nach_Reed–Solomon–Codierung|Fehlerkorrektur nach Reed–Solomon–Codierung]] Anwendung findet, eine Fehlentscheidung $(\underline{z} \ne \underline{c})$ ausgeschlossen ⇒ Blockfehlerwahrscheinlichkeit ${\rm Pr}(\underline{z}\ne\underline{c}) = 0$ ⇒ ${\rm Pr}(\underline{v}\ne\underline{u}) = 0$. | ||
| + | *Das rekonstruierte Informationswort ergibt sich gemäß dem Blockschaltbild (gelbe Hinterlegung) zu $\underline{v} = {\rm enc}^{-1}(\underline{z})$. | ||
| + | *Mit der Generatormatrix $\boldsymbol{\rm G}$ kann hierfür auch geschrieben werden: | ||
| + | |||
| + | ::<math>\underline {c} = \underline {u} \cdot { \boldsymbol{\rm G}} | ||
\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {z} = \underline {\upsilon} \cdot { \boldsymbol{\rm G}} | \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {z} = \underline {\upsilon} \cdot { \boldsymbol{\rm G}} | ||
\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {\upsilon} = \underline {z} \cdot { \boldsymbol{\rm G}}^{\rm T} | \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {\upsilon} = \underline {z} \cdot { \boldsymbol{\rm G}}^{\rm T} | ||
| Zeile 45: | Zeile 59: | ||
== Vorgehensweise am Beispiel des RSC (7, 3, 5)<sub>8</sub> == | == Vorgehensweise am Beispiel des RSC (7, 3, 5)<sub>8</sub> == | ||
<br> | <br> | ||
| − | Um die | + | Um die Reed–Solomon–Decodierung beim Auslöschungskanal so einfach wie möglich darstellen zu können, gehen wir von einer konkreten Aufgabenstellung aus: |
| − | + | ||
| + | Verwendet wird ein Reed–Solomon–Code mit den Parametern $n= 7$, $k= 3$ und $q= 2^3 = 8$. | ||
| + | |||
| + | Somit gilt für das Informationswort $\underline{u}$ und das Codewort $\underline{c}$: | ||
::<math>\underline {u} = (u_0, u_1, u_2) \hspace{0.05cm},\hspace{0.15cm} | ::<math>\underline {u} = (u_0, u_1, u_2) \hspace{0.05cm},\hspace{0.15cm} | ||
\underline {c} = (c_0, c_1, c_2,c_3,c_4,c_5,c_6)\hspace{0.05cm},\hspace{0.15cm} | \underline {c} = (c_0, c_1, c_2,c_3,c_4,c_5,c_6)\hspace{0.05cm},\hspace{0.15cm} | ||
| − | u_i, c_i \in {\rm GF}(2^3) = \{0, 1, \alpha, \alpha^2, ... , \alpha^6\} | + | u_i, c_i \in {\rm GF}(2^3) = \{0, 1, \alpha, \alpha^2, \text{...}\hspace{0.05cm} , \alpha^6\} |
\hspace{0.05cm},</math> | \hspace{0.05cm},</math> | ||
| + | |||
| + | und die Prüfmatrix $\boldsymbol{\rm H}$ lautet: | ||
::<math>{ \boldsymbol{\rm H}} = | ::<math>{ \boldsymbol{\rm H}} = | ||
| Zeile 61: | Zeile 80: | ||
\end{pmatrix}\hspace{0.05cm}. </math> | \end{pmatrix}\hspace{0.05cm}. </math> | ||
| − | + | Beispielhaft wird hier vom Empfangsvektor $\underline {y} = (\alpha, \hspace{0.03cm} 1, \hspace{0.03cm}{\rm E}, \hspace{0.03cm}{\rm E}, \hspace{0.03cm}\alpha^2,{\rm E}, \hspace{0.03cm}\alpha^5)$ ausgegangen. Dann gilt: | |
| + | *Da der Auslöschungskanal keine Fehler produziert, sind dem Decoder vier der Codesymbole bekannt: | ||
::<math>c_0 = \alpha^1 \hspace{0.05cm},\hspace{0.2cm} | ::<math>c_0 = \alpha^1 \hspace{0.05cm},\hspace{0.2cm} | ||
| Zeile 69: | Zeile 89: | ||
\hspace{0.05cm}.</math> | \hspace{0.05cm}.</math> | ||
| − | *Es ist offensichtlich, dass der Block „Codewortfinder” – im Blockschaltbild mit CWF bezeichnet – einen Vektor der Form | + | *Es ist offensichtlich, dass der Block „Codewortfinder” – im Blockschaltbild mit '''CWF''' bezeichnet – einen Vektor der Form $\underline {z} = (c_0, \hspace{0.03cm}c_1, \hspace{0.03cm}z_2, \hspace{0.03cm}z_3,\hspace{0.03cm}c_4,\hspace{0.03cm}z_5,\hspace{0.03cm}c_6)$ liefern soll mit $z_2,\hspace{0.03cm}z_3,\hspace{0.03cm}z_5 \in \rm GF(2^3)$.<br> |
| − | *Da das vom Decoder gefundene Codewort | + | *Da das vom Decoder gefundene Codewort $\underline {z}$ aber auch ein gültiges Reed–Solomon–Codewort sein soll ⇒ $\underline {z} ∈ \mathcal{C}_{\rm RS}$, muss ebenso gelten: |
::<math>{ \boldsymbol{\rm H}} \cdot \underline {z}^{\rm T} = \underline {0}^{\rm T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ::<math>{ \boldsymbol{\rm H}} \cdot \underline {z}^{\rm T} = \underline {0}^{\rm T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
| Zeile 96: | Zeile 116: | ||
\hspace{0.05cm}. </math> | \hspace{0.05cm}. </math> | ||
| − | *Daraus ergeben sich vier Gleichungen für die Unbekannten | + | *Daraus ergeben sich vier Gleichungen für die Unbekannten $z_2$, $z_3$ und $z_5$. Bei eindeutiger Lösung – und nur bei einer solchen – ist die Decodierung erfolgreich und man kann dann mit Sicherheit sagen, dass tatsächlich $\underline {c} = \underline {z} $ gesendet wurde.<br><br> |
| + | |||
| − | |||
== Lösung der Matrixgleichungen am Beispiel des RSC (7, 3, 5)<sub>8</sub> == | == Lösung der Matrixgleichungen am Beispiel des RSC (7, 3, 5)<sub>8</sub> == | ||
<br> | <br> | ||
| − | Gefunden werden muss also das zulässige Codewort | + | Gefunden werden muss also das zulässige Codewort $\underline {z}$, das die Bestimmungsgleichung $\boldsymbol{\rm H} \cdot \underline {z}^{\rm T} $ erfüllt. Zweckmäßigerweise spalten wir dazu den Vektor $\underline {z}$ in zwei Teilvektoren auf, nämlich in |
| − | *den Vektor | + | *den Vektor $\underline {z}_{\rm E} = (z_2, z_3, z_5)$ der ausgelöschten Symbole (Index „$\rm E$” für <i>Erasures</i> ),<br> |
| − | *den Vektor | + | *den Vektor $\underline {z}_{\rm K} = (c_0, c_1,c_4, c_6)$ der bekannten Symbole (Index „$\rm K$” für <i>Korrekt</i> ).<br><br> |
| − | Mit den zugehörigen Teilmatrizen (jeweils mit | + | Mit den zugehörigen Teilmatrizen (jeweils mit $n-k = 4$ Zeilen) |
| − | :<math>{ \boldsymbol{\rm H}}_{\rm E} = | + | ::<math>{ \boldsymbol{\rm H}}_{\rm E} = |
\begin{pmatrix} | \begin{pmatrix} | ||
\alpha^2 & \alpha^3 & \alpha^5 \\ | \alpha^2 & \alpha^3 & \alpha^5 \\ | ||
| Zeile 126: | Zeile 146: | ||
lautet somit die Bestimmungsgleichung: | lautet somit die Bestimmungsgleichung: | ||
| − | :<math>{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} + | + | ::<math>{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} + |
{ \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} | { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} | ||
= \underline {0}^{\rm T} \hspace{0.5cm} \Rightarrow \hspace{0.5cm} | = \underline {0}^{\rm T} \hspace{0.5cm} \Rightarrow \hspace{0.5cm} | ||
| Zeile 132: | Zeile 152: | ||
{ \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T}\hspace{0.05cm}. </math> | { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T}\hspace{0.05cm}. </math> | ||
| − | Da für alle Elemente | + | *Da für alle Elemente $z_i ∈ {\rm GF}(2^m)$ die [[Kanalcodierung/Einige_Grundlagen_der_Algebra#Definition_eines_Galoisfeldes |additive Inverse]] ${\rm Inv_A}(z_i)= (- z_i) = z_i$ ist, gilt in gleicher Weise |
| − | :<math>{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} = | + | ::<math>{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} = |
{ \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} = | { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} = | ||
\begin{pmatrix} | \begin{pmatrix} | ||
| Zeile 147: | Zeile 167: | ||
\alpha^{2}\\ | \alpha^{2}\\ | ||
\alpha^{6} | \alpha^{6} | ||
| − | \end{pmatrix} = \hspace{0. | + | \end{pmatrix} = \hspace{0.45cm}... \hspace{0.45cm}= |
\begin{pmatrix} | \begin{pmatrix} | ||
\alpha^3\\ | \alpha^3\\ | ||
| Zeile 156: | Zeile 176: | ||
\hspace{0.05cm}.</math> | \hspace{0.05cm}.</math> | ||
| − | Die rechte Gleichungsseite ergibt für das betrachtete Beispiel ⇒ | + | *Die rechte Gleichungsseite ergibt sich für das betrachtete Beispiel ⇒ $\underline {z}_{\rm K} = (c_0, c_1,c_4, c_6)$ und basiert auf dem Polynom $p(x) = x^3 + x +1$, das zu folgenden Potenzen $($in $\alpha)$ führt: |
| − | :<math>\alpha^3 | + | ::<math>\alpha^3 =\alpha + 1\hspace{0.05cm}, |
| − | \hspace{0. | + | \hspace{0.3cm} \alpha^4 = \alpha^2 + \alpha\hspace{0.05cm}, |
| − | \hspace{0. | + | \hspace{0.3cm} \alpha^5 = \alpha^2 + \alpha + 1\hspace{0.05cm}, |
| − | \hspace{0. | + | \hspace{0.3cm} \alpha^6 = \alpha^2 + 1\hspace{0.05cm}, |
| − | + | \hspace{0.3cm} \alpha^7 \hspace{-0.15cm} = \hspace{-0.15cm} 1\hspace{0.05cm}, | |
| − | \hspace{ | + | \hspace{0.3cm} \alpha^8 = \alpha^1 \hspace{0.05cm}, |
| − | \hspace{ | + | \hspace{0.3cm} \alpha^9 = \alpha^2 \hspace{0.05cm}, |
| − | \hspace{ | + | \hspace{0.3cm} \alpha^{10} = \alpha^3 = \alpha + 1\hspace{0.05cm},\hspace{0.1cm} \text{...}</math> |
| − | Damit lautet die Matrizengleichung zur Bestimmung des gesuchten Vektors | + | *Damit lautet die Matrizengleichung zur Bestimmung des gesuchten Vektors $\underline {z}_{\rm E}$: |
| − | :<math>\begin{pmatrix} | + | ::<math>\begin{pmatrix} |
\alpha^2 & \alpha^3 & \alpha^5 \\ | \alpha^2 & \alpha^3 & \alpha^5 \\ | ||
\alpha^4 & \alpha^6 & \alpha^{3} \\ | \alpha^4 & \alpha^6 & \alpha^{3} \\ | ||
| Zeile 188: | Zeile 208: | ||
\hspace{0.05cm}. </math> | \hspace{0.05cm}. </math> | ||
| − | Löst man diese Matrizengleichung (am einfachsten per Programm), so erhält man | + | *Löst man diese Matrizengleichung (am einfachsten per Programm), so erhält man |
| − | :<math>z_2 = \alpha^2\hspace{0.05cm},\hspace{0.25cm}z_3 = \alpha^1\hspace{0.05cm},\hspace{0.25cm}z_5 = \alpha^5 | + | ::<math>z_2 = \alpha^2\hspace{0.05cm},\hspace{0.25cm}z_3 = \alpha^1\hspace{0.05cm},\hspace{0.25cm}z_5 = \alpha^5 |
\hspace{0.5cm} \Rightarrow \hspace{0.5cm}\underline {z} = \left ( \hspace{0.05cm} \alpha^1, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^5, \hspace{0.05cm}\alpha^5 \hspace{0.05cm}\right ) | \hspace{0.5cm} \Rightarrow \hspace{0.5cm}\underline {z} = \left ( \hspace{0.05cm} \alpha^1, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^5, \hspace{0.05cm}\alpha^5 \hspace{0.05cm}\right ) | ||
\hspace{0.05cm}.</math> | \hspace{0.05cm}.</math> | ||
| − | Das Ergebnis ist richtig, wie die folgenden Kontrollrechnungen zeigen: | + | *Das Ergebnis ist richtig, wie die folgenden Kontrollrechnungen zeigen: |
| − | :<math>\alpha^2 \cdot \alpha^2 + \alpha^3 \cdot \alpha^1 + \alpha^5 \cdot \alpha^5 | + | ::<math>\alpha^2 \cdot \alpha^2 + \alpha^3 \cdot \alpha^1 + \alpha^5 \cdot \alpha^5 = |
\alpha^4 + \alpha^4 + \alpha^{10} = \alpha^{10} = \alpha^3\hspace{0.05cm},</math> | \alpha^4 + \alpha^4 + \alpha^{10} = \alpha^{10} = \alpha^3\hspace{0.05cm},</math> | ||
| − | :<math>\alpha^4 \cdot \alpha^2 + \alpha^6 \cdot \alpha^1 + \alpha^3 \cdot \alpha^5 | + | ::<math>\alpha^4 \cdot \alpha^2 + \alpha^6 \cdot \alpha^1 + \alpha^3 \cdot \alpha^5 = |
(\alpha^2 + 1) + (1) + (\alpha) = \alpha^{2} + \alpha = \alpha^4\hspace{0.05cm},</math> | (\alpha^2 + 1) + (1) + (\alpha) = \alpha^{2} + \alpha = \alpha^4\hspace{0.05cm},</math> | ||
| − | :<math>\alpha^6 \cdot \alpha^2 + \alpha^2 \cdot \alpha^1 + \alpha^1 \cdot \alpha^5 | + | ::<math>\alpha^6 \cdot \alpha^2 + \alpha^2 \cdot \alpha^1 + \alpha^1 \cdot \alpha^5 = |
(\alpha) + (\alpha + 1) + (\alpha^2 + 1) = \alpha^{2} \hspace{0.05cm},</math> | (\alpha) + (\alpha + 1) + (\alpha^2 + 1) = \alpha^{2} \hspace{0.05cm},</math> | ||
| − | :<math>\alpha^1 \cdot \alpha^2 + \alpha^5 \cdot \alpha^1 + \alpha^6 \cdot \alpha^5 | + | ::<math>\alpha^1 \cdot \alpha^2 + \alpha^5 \cdot \alpha^1 + \alpha^6 \cdot \alpha^5 = |
(\alpha + 1) + (\alpha^2 + 1) + (\alpha^2 + \alpha) = 0\hspace{0.05cm}.</math> | (\alpha + 1) + (\alpha^2 + 1) + (\alpha^2 + \alpha) = 0\hspace{0.05cm}.</math> | ||
| − | Das zugehörige Informationswort erhält man mit der [ | + | *Das zugehörige Informationswort erhält man mit der [[Kanalcodierung/Allgemeine_Beschreibung_linearer_Blockcodes#Codefestlegung_durch_die_Generatormatrix| Generatormatrix]] $\boldsymbol{\rm G}$ zu $\underline {v} = \underline {z} \cdot \boldsymbol{\rm G}^{\rm T} = (\alpha^1,\hspace{0.05cm}1,\hspace{0.05cm}\alpha^3)$.<br> |
| − | == Aufgaben == | + | == Aufgaben zum Kapitel == |
<br> | <br> | ||
| − | [[Aufgaben:2. | + | [[Aufgaben:2.11_RS–Decodierung_nach_„Erasures”|Aufgabe 2.11: RS–Decodierung nach „Erasures”]] |
| − | [[ | + | [[Aufgaben:2.11Z_Erasure–Kanal_für_Symbole|Aufgabe 2.11Z: Erasure–Kanal für Symbole]] |
{{Display}} | {{Display}} | ||
Aktuelle Version vom 20. Oktober 2022, 16:28 Uhr
Inhaltsverzeichnis
Blockschaltbild und Voraussetzungen zur Reed–Solomon–Fehlererkennung
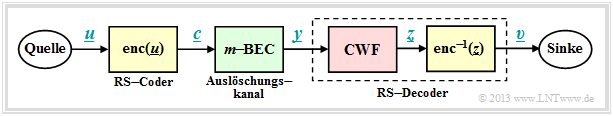
Im Kapitel "Decodierung beim Binary Erasure Channel" wurde für die binären Blockcodes gezeigt, welche Berechnungen der Decoder ausführen muss, um aus einem unvollständigen Empfangswort $\underline{y}$ das gesendete Codewort $\underline{x}$ bestmöglich decodieren zu können. Im Reed–Solomon–Kapitel haben wir $\underline{x}$ in $\underline{c}$ umbenannt.
- Zugrunde gelegt wird hier wieder das "BEC–Kanalmodell" ("Binary Erasure Channel"), das ein unsicheres Bit als "Erasure" $\rm E$ ("Auslöschung") markiert.
- Im Gegensatz zu "BSC-Modell" ("Binary Symmetric Channel") und "AWGN-Modell" ("Additive White Gaussian Noise") sind hier Bitfehler $(y_i ≠ c_i)$ ausgeschlossen.
Jedes Bit eines Empfangswortes $\underline{y}$
- stimmt also mit dem entsprechenden Bit des Codewortes überein $(y_i = c_i)$, oder
- ist bereits als Auslöschung markiert $(y_i = \rm E)$.
Die Grafik zeigt das Blockschaltbild, das sich von dem Modell im Kapitel "Decodierung linearer Blockcodes" geringfügig unterscheidet:
- Da Reed–Solomon–Codes lineare Blockcodes sind, stehen auch hier Informationswort $\underline{u}$ und Codewort $\underline{c}$ über die Generatormatrix $\boldsymbol{\rm G}$ und die folgende Gleichung in Zusammenhang:
- \[\underline {c} = {\rm enc}(\underline {u}) = \underline {u} \cdot { \boldsymbol{\rm G}} \hspace{0.6cm} {\rm mit} \hspace{0.6cm}\underline {u} = (u_0, u_1,\hspace{0.05cm}\text{ ... }\hspace{0.1cm}, u_i, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, u_{k-1})\hspace{0.05cm}, \hspace{0.2cm} \underline {c} = (c_0, c_1, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, c_i, \hspace{0.05cm}\text{ ... }\hspace{0.1cm}, c_{n-1}) \hspace{0.05cm}.\]
- Für die einzelnen Symbole von Informationsblock und Codewort gilt bei Reed–Solomon–Codierung:
- \[u_i \in {\rm GF}(q)\hspace{0.05cm},\hspace{0.2cm}c_i \in {\rm GF}(q)\hspace{0.6cm}{\rm mit}\hspace{0.6cm} q = n+1 = 2^m \hspace{0.3cm} \Rightarrow \hspace{0.3cm} n = 2^m - 1\hspace{0.05cm}. \]
- Jedes Codesymbol $c_i$ wird somit mit $m ≥ 2$ Binärsymbolen ("Bit") dargestellt. Zum Vergleich: Für die binären Blockcodes gilt $q=2$, $m=1$ und die Codewortlänge $n$ ist frei wählbar.
- Bei Codierung auf Symbolebene muss das "BEC–Modell" zum "$m$–BEC–Modell" erweitert werden.
- Mit der Wahrscheinlichkeit $\lambda_m ≈ m \cdot\lambda$ wird ein Codesymbol $c_i$ ausgelöscht $(y_i = \rm E)$ und es gilt ${\rm Pr}(y_i = c_i) = 1 - \lambda_m$.
- Näheres zur Umrechnung der beiden Modelle finden Sie in der Aufgabe 2.11Z.
- Näheres zur Umrechnung der beiden Modelle finden Sie in der Aufgabe 2.11Z.
Im Folgenden beschäftigen wir uns nur mit dem Block "Codewortfinder" $\rm (CWF)$, der aus dem Empfangsvektor $\underline{y}$ den Vektor $\underline{z} ∈ \mathcal{C}_{\rm RS}$ gewinnt:
- Falls die Anzahl $e$ der Auslöschungen im Vektor $\underline{y}$ hinreichend klein ist, lässt sich das gesamte Codewort mit Sicherheit $(\underline{z}=\underline{c})$ finden.
- Sind zuviele Symbole des Empfangswortes $\underline{y}$ ausgelöscht, meldet der Decoder, dass dieses Wort nicht decodierbar ist. Eventuell muss dann diese Sequenz noch einmal gesendet werden.
Beim Auslöschungskanal ($m$–BEC) ist im Gegensatz zum $m$–BSC, der im Kapitel Fehlerkorrektur nach Reed–Solomon–Codierung Anwendung findet, eine Fehlentscheidung $(\underline{z} \ne \underline{c})$ ausgeschlossen ⇒ Blockfehlerwahrscheinlichkeit ${\rm Pr}(\underline{z}\ne\underline{c}) = 0$ ⇒ ${\rm Pr}(\underline{v}\ne\underline{u}) = 0$.
- Das rekonstruierte Informationswort ergibt sich gemäß dem Blockschaltbild (gelbe Hinterlegung) zu $\underline{v} = {\rm enc}^{-1}(\underline{z})$.
- Mit der Generatormatrix $\boldsymbol{\rm G}$ kann hierfür auch geschrieben werden:
- \[\underline {c} = \underline {u} \cdot { \boldsymbol{\rm G}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {z} = \underline {\upsilon} \cdot { \boldsymbol{\rm G}} \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline {\upsilon} = \underline {z} \cdot { \boldsymbol{\rm G}}^{\rm T} \hspace{0.05cm}. \]
Vorgehensweise am Beispiel des RSC (7, 3, 5)8
Um die Reed–Solomon–Decodierung beim Auslöschungskanal so einfach wie möglich darstellen zu können, gehen wir von einer konkreten Aufgabenstellung aus:
Verwendet wird ein Reed–Solomon–Code mit den Parametern $n= 7$, $k= 3$ und $q= 2^3 = 8$.
Somit gilt für das Informationswort $\underline{u}$ und das Codewort $\underline{c}$:
- \[\underline {u} = (u_0, u_1, u_2) \hspace{0.05cm},\hspace{0.15cm} \underline {c} = (c_0, c_1, c_2,c_3,c_4,c_5,c_6)\hspace{0.05cm},\hspace{0.15cm} u_i, c_i \in {\rm GF}(2^3) = \{0, 1, \alpha, \alpha^2, \text{...}\hspace{0.05cm} , \alpha^6\} \hspace{0.05cm},\]
und die Prüfmatrix $\boldsymbol{\rm H}$ lautet:
- \[{ \boldsymbol{\rm H}} = \begin{pmatrix} 1 & \alpha^1 & \alpha^2 & \alpha^3 & \alpha^4 & \alpha^5 & \alpha^6\\ 1 & \alpha^2 & \alpha^4 & \alpha^6 & \alpha^1 & \alpha^{3} & \alpha^{5}\\ 1 & \alpha^3 & \alpha^6 & \alpha^2 & \alpha^{5} & \alpha^{1} & \alpha^{4}\\ 1 & \alpha^4 & \alpha^1 & \alpha^{5} & \alpha^{2} & \alpha^{6} & \alpha^{3} \end{pmatrix}\hspace{0.05cm}. \]
Beispielhaft wird hier vom Empfangsvektor $\underline {y} = (\alpha, \hspace{0.03cm} 1, \hspace{0.03cm}{\rm E}, \hspace{0.03cm}{\rm E}, \hspace{0.03cm}\alpha^2,{\rm E}, \hspace{0.03cm}\alpha^5)$ ausgegangen. Dann gilt:
- Da der Auslöschungskanal keine Fehler produziert, sind dem Decoder vier der Codesymbole bekannt:
- \[c_0 = \alpha^1 \hspace{0.05cm},\hspace{0.2cm} c_1 = 1 \hspace{0.05cm},\hspace{0.2cm} c_4 = \alpha^2 \hspace{0.05cm},\hspace{0.2cm} c_6 = \alpha^5 \hspace{0.05cm}.\]
- Es ist offensichtlich, dass der Block „Codewortfinder” – im Blockschaltbild mit CWF bezeichnet – einen Vektor der Form $\underline {z} = (c_0, \hspace{0.03cm}c_1, \hspace{0.03cm}z_2, \hspace{0.03cm}z_3,\hspace{0.03cm}c_4,\hspace{0.03cm}z_5,\hspace{0.03cm}c_6)$ liefern soll mit $z_2,\hspace{0.03cm}z_3,\hspace{0.03cm}z_5 \in \rm GF(2^3)$.
- Da das vom Decoder gefundene Codewort $\underline {z}$ aber auch ein gültiges Reed–Solomon–Codewort sein soll ⇒ $\underline {z} ∈ \mathcal{C}_{\rm RS}$, muss ebenso gelten:
- \[{ \boldsymbol{\rm H}} \cdot \underline {z}^{\rm T} = \underline {0}^{\rm T} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \begin{pmatrix} 1 & \alpha^1 & \alpha^2 & \alpha^3 & \alpha^4 & \alpha^5 & \alpha^6\\ 1 & \alpha^2 & \alpha^4 & \alpha^6 & \alpha^1 & \alpha^{3} & \alpha^{5}\\ 1 & \alpha^3 & \alpha^6 & \alpha^2 & \alpha^{5} & \alpha^{1} & \alpha^{4}\\ 1 & \alpha^4 & \alpha^1 & \alpha^{5} & \alpha^{2} & \alpha^{6} & \alpha^{3} \end{pmatrix} \cdot \begin{pmatrix} c_0\\ c_1\\ z_2\\ z_3\\ c_4\\ z_5\\ c_6 \end{pmatrix} = \begin{pmatrix} 0\\ 0\\ 0\\ 0 \end{pmatrix} \hspace{0.05cm}. \]
- Daraus ergeben sich vier Gleichungen für die Unbekannten $z_2$, $z_3$ und $z_5$. Bei eindeutiger Lösung – und nur bei einer solchen – ist die Decodierung erfolgreich und man kann dann mit Sicherheit sagen, dass tatsächlich $\underline {c} = \underline {z} $ gesendet wurde.
Lösung der Matrixgleichungen am Beispiel des RSC (7, 3, 5)8
Gefunden werden muss also das zulässige Codewort $\underline {z}$, das die Bestimmungsgleichung $\boldsymbol{\rm H} \cdot \underline {z}^{\rm T} $ erfüllt. Zweckmäßigerweise spalten wir dazu den Vektor $\underline {z}$ in zwei Teilvektoren auf, nämlich in
- den Vektor $\underline {z}_{\rm E} = (z_2, z_3, z_5)$ der ausgelöschten Symbole (Index „$\rm E$” für Erasures ),
- den Vektor $\underline {z}_{\rm K} = (c_0, c_1,c_4, c_6)$ der bekannten Symbole (Index „$\rm K$” für Korrekt ).
Mit den zugehörigen Teilmatrizen (jeweils mit $n-k = 4$ Zeilen)
- \[{ \boldsymbol{\rm H}}_{\rm E} = \begin{pmatrix} \alpha^2 & \alpha^3 & \alpha^5 \\ \alpha^4 & \alpha^6 & \alpha^{3} \\ \alpha^6 & \alpha^2 & \alpha^{1} \\ \alpha^1 & \alpha^{5} & \alpha^{6} \end{pmatrix} \hspace{0.05cm},\hspace{0.4cm} { \boldsymbol{\rm H}}_{\rm K} \begin{pmatrix} 1 & \alpha^1 & \alpha^4 & \alpha^6\\ 1 & \alpha^2 & \alpha^1 & \alpha^{5}\\ 1 & \alpha^3 & \alpha^{5} & \alpha^{4}\\ 1 & \alpha^4 & \alpha^{2} & \alpha^{3} \end{pmatrix}\]
lautet somit die Bestimmungsgleichung:
- \[{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} + { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} = \underline {0}^{\rm T} \hspace{0.5cm} \Rightarrow \hspace{0.5cm} { \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} = - { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T}\hspace{0.05cm}. \]
- Da für alle Elemente $z_i ∈ {\rm GF}(2^m)$ die additive Inverse ${\rm Inv_A}(z_i)= (- z_i) = z_i$ ist, gilt in gleicher Weise
- \[{ \boldsymbol{\rm H}}_{\rm E} \cdot \underline {z}_{\rm E}^{\rm T} = { \boldsymbol{\rm H}}_{\rm K} \cdot \underline {z}_{\rm K}^{\rm T} = \begin{pmatrix} 1 & \alpha^1 & \alpha^4 & \alpha^6\\ 1 & \alpha^2 & \alpha^1 & \alpha^{5}\\ 1 & \alpha^3 & \alpha^{5} & \alpha^{4}\\ 1 & \alpha^4 & \alpha^{2} & \alpha^{3} \end{pmatrix} \cdot \begin{pmatrix} \alpha^1\\ 1\\ \alpha^{2}\\ \alpha^{6} \end{pmatrix} = \hspace{0.45cm}... \hspace{0.45cm}= \begin{pmatrix} \alpha^3\\ \alpha^{4}\\ \alpha^{2}\\ 0 \end{pmatrix} \hspace{0.05cm}.\]
- Die rechte Gleichungsseite ergibt sich für das betrachtete Beispiel ⇒ $\underline {z}_{\rm K} = (c_0, c_1,c_4, c_6)$ und basiert auf dem Polynom $p(x) = x^3 + x +1$, das zu folgenden Potenzen $($in $\alpha)$ führt:
- \[\alpha^3 =\alpha + 1\hspace{0.05cm}, \hspace{0.3cm} \alpha^4 = \alpha^2 + \alpha\hspace{0.05cm}, \hspace{0.3cm} \alpha^5 = \alpha^2 + \alpha + 1\hspace{0.05cm}, \hspace{0.3cm} \alpha^6 = \alpha^2 + 1\hspace{0.05cm}, \hspace{0.3cm} \alpha^7 \hspace{-0.15cm} = \hspace{-0.15cm} 1\hspace{0.05cm}, \hspace{0.3cm} \alpha^8 = \alpha^1 \hspace{0.05cm}, \hspace{0.3cm} \alpha^9 = \alpha^2 \hspace{0.05cm}, \hspace{0.3cm} \alpha^{10} = \alpha^3 = \alpha + 1\hspace{0.05cm},\hspace{0.1cm} \text{...}\]
- Damit lautet die Matrizengleichung zur Bestimmung des gesuchten Vektors $\underline {z}_{\rm E}$:
- \[\begin{pmatrix} \alpha^2 & \alpha^3 & \alpha^5 \\ \alpha^4 & \alpha^6 & \alpha^{3} \\ \alpha^6 & \alpha^2 & \alpha^{1} \\ \alpha^1 & \alpha^{5} & \alpha^{6} \end{pmatrix} \cdot \begin{pmatrix} z_2\\ z_3\\ z_5 \end{pmatrix} \stackrel{!}{=} \begin{pmatrix} \alpha^3\\ \alpha^{4}\\ \alpha^{2}\\ 0 \end{pmatrix} \hspace{0.05cm}. \]
- Löst man diese Matrizengleichung (am einfachsten per Programm), so erhält man
- \[z_2 = \alpha^2\hspace{0.05cm},\hspace{0.25cm}z_3 = \alpha^1\hspace{0.05cm},\hspace{0.25cm}z_5 = \alpha^5 \hspace{0.5cm} \Rightarrow \hspace{0.5cm}\underline {z} = \left ( \hspace{0.05cm} \alpha^1, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^1, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}\alpha^5, \hspace{0.05cm}\alpha^5 \hspace{0.05cm}\right ) \hspace{0.05cm}.\]
- Das Ergebnis ist richtig, wie die folgenden Kontrollrechnungen zeigen:
- \[\alpha^2 \cdot \alpha^2 + \alpha^3 \cdot \alpha^1 + \alpha^5 \cdot \alpha^5 = \alpha^4 + \alpha^4 + \alpha^{10} = \alpha^{10} = \alpha^3\hspace{0.05cm},\]
- \[\alpha^4 \cdot \alpha^2 + \alpha^6 \cdot \alpha^1 + \alpha^3 \cdot \alpha^5 = (\alpha^2 + 1) + (1) + (\alpha) = \alpha^{2} + \alpha = \alpha^4\hspace{0.05cm},\]
- \[\alpha^6 \cdot \alpha^2 + \alpha^2 \cdot \alpha^1 + \alpha^1 \cdot \alpha^5 = (\alpha) + (\alpha + 1) + (\alpha^2 + 1) = \alpha^{2} \hspace{0.05cm},\]
- \[\alpha^1 \cdot \alpha^2 + \alpha^5 \cdot \alpha^1 + \alpha^6 \cdot \alpha^5 = (\alpha + 1) + (\alpha^2 + 1) + (\alpha^2 + \alpha) = 0\hspace{0.05cm}.\]
- Das zugehörige Informationswort erhält man mit der Generatormatrix $\boldsymbol{\rm G}$ zu $\underline {v} = \underline {z} \cdot \boldsymbol{\rm G}^{\rm T} = (\alpha^1,\hspace{0.05cm}1,\hspace{0.05cm}\alpha^3)$.
Aufgaben zum Kapitel
Aufgabe 2.11: RS–Decodierung nach „Erasures”
Aufgabe 2.11Z: Erasure–Kanal für Symbole