Aufgaben:Aufgabe 2.3Z: Schwingungsparameter: Unterschied zwischen den Versionen
Aus LNTwww
Nabil (Diskussion | Beiträge) (Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Signaldarstellung/Harmonische Schwingung }} right| Jede harmonische Schwingung kann auch in der Form…“) |
|||
| (12 dazwischenliegende Versionen von 2 Benutzern werden nicht angezeigt) | |||
| Zeile 3: | Zeile 3: | ||
}} | }} | ||
| − | [[Datei:P_ID316__Sig_Z_2_3.png|right|]] | + | [[Datei:P_ID316__Sig_Z_2_3.png|right|frame|Definitionen von $x_0$, $t_1$ und $t_2$]] |
Jede harmonische Schwingung kann auch in der Form | Jede harmonische Schwingung kann auch in der Form | ||
:$$x(t)=C\cdot\cos\bigg(2\pi \cdot \frac{t-\tau}{T_0}\bigg)$$ | :$$x(t)=C\cdot\cos\bigg(2\pi \cdot \frac{t-\tau}{T_0}\bigg)$$ | ||
geschrieben werden. Die Schwingung ist somit durch drei Parameter vollständig bestimmt: | geschrieben werden. Die Schwingung ist somit durch drei Parameter vollständig bestimmt: | ||
| − | :* die Amplitude $C$, | + | :* die Amplitude $C$, |
| − | :* die Periodendauer $T_0$, | + | :* die Periodendauer $T_0$, |
| − | :* die Verschiebung $\tau$ gegenüber einem Cosinussignal. | + | :* die Verschiebung $\tau$ gegenüber einem Cosinussignal. |
| − | Eine zweite Darstellungsform lautet mit der Grundfrequenz $f_0$ und der Phase $\varphi$: | + | |
| + | Eine zweite Darstellungsform lautet mit der Grundfrequenz $f_0$ und der Phase $\varphi$: | ||
:$$x(t)=C \cdot\cos(2\pi f_0t-\varphi).$$ | :$$x(t)=C \cdot\cos(2\pi f_0t-\varphi).$$ | ||
Von einer harmonischen Schwingung ist nun bekannt, dass | Von einer harmonischen Schwingung ist nun bekannt, dass | ||
| − | :* das erste Signalmaximum bei $t_1 = 2 \text{ms}$ auftritt, | + | :* das erste Signalmaximum bei $t_1 = 2 \,\text{ms}$ auftritt, |
| + | |||
| + | :* das zweite Signalmaximum bei $t_2 = 14 \,\text{ms}$ auftritt, | ||
| + | |||
| + | :* der Wert $x_0 ={x(t = 0)} = 3 \,\text{V}$ ist. | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| − | |||
| − | :* | + | ''Hinweis:'' |
| + | *Die Aufgabe gehört zum Kapitel [[Signaldarstellung/Harmonische_Schwingung|Harmonische Schwingung]]. | ||
| + | |||
| − | |||
| Zeile 30: | Zeile 40: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {Wie groß ist die Periodendauer | + | {Wie groß ist die Periodendauer $T_0$ und die Grundfrequenz $f_0$? |
|type="{}"} | |type="{}"} | ||
| − | $T_0$ | + | $T_0\hspace{0.2cm} = \ $ { 12 3% } $\text{ms}$ |
| − | $f_0$ | + | $f_0\hspace{0.2cm} = \ $ { 83.33 3% } $\text{Hz}$ |
| − | {Welchen Wert haben hier die Verschiebung $\tau$ und die Phase $\varphi$ (in $\text{Grad}$ | + | {Welchen Wert haben hier die Verschiebung $\tau$ und die Phase $\varphi$ $($in $\text{Grad})$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\tau$ | + | $\tau\hspace{0.25cm} = \ $ { 2 3% } $\text{ms}$ |
| − | $\varphi$ | + | $\varphi\hspace{0.2cm} = \ $ { 60 3% } $\text{Grad}$ |
{Wie groß ist die Amplitude der harmonischen Schwingung? | {Wie groß ist die Amplitude der harmonischen Schwingung? | ||
|type="{}"} | |type="{}"} | ||
| − | $ | + | ${C}\ = \ $ { 6 3% } $\text{V}$ |
| − | {Wie lautet das Spektrum? Welches Gewicht hat die Spektrallinie bei $+f_0$? | + | {Wie lautet das Spektrum $X(f)$? Welches Gewicht hat die Spektrallinie bei $+f_0$ ? |
|type="{}"} | |type="{}"} | ||
| − | $\text{Re}[X(f = f_0)]$ | + | $\text{Re}\big[X(f = f_0)\big]\ = \ $ { 1.5 3% } $\text{V}$ |
| − | $\text{Im}[X(f = f_0)] | + | $\text{Im}\big[X(f = f_0)\big] \ = \ $ { -2.65--2.55 } $\text{V}$ |
| Zeile 58: | Zeile 68: | ||
===Musterlösung=== | ===Musterlösung=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''1 | + | '''(1)''' Es gilt $T_0 = t_2 - t_1 = 12\, \text{ms}$ und $f_0 = 1/T_0 \hspace{0.15cm} \underline{\approx 83.33\, \text{Hz}}$. |
| + | |||
| + | |||
| − | '''2 | + | '''(2)''' Die Verschiebung beträgt $\tau \hspace{0.1cm} \underline{= 2\, \text{ms}}$ und die Phase ist $\varphi = 2\pi \cdot \tau/T_0 = \pi/3$ entsprechend $\varphi =\hspace{0.15cm} \underline{60^{\circ}}$. |
| − | '''3 | + | |
| − | :$$x_0=x(t=0)=C\cdot\cos(-60\,^\circ)= | + | |
| + | '''(3)''' Aus dem Wert zum Zeitpunkt $t = 0$ folgt für die Amplitude ${C}$: | ||
| + | :$$x_0=x(t=0)=C\cdot\cos(-60\,^\circ)={C}/{2}=\rm 3\,V | ||
\hspace{0.3 cm} \Rightarrow \hspace{0.3 cm}\hspace{0.15cm}\underline{\it C=\rm 6\,V}.$$ | \hspace{0.3 cm} \Rightarrow \hspace{0.3 cm}\hspace{0.15cm}\underline{\it C=\rm 6\,V}.$$ | ||
| − | '''4 | + | |
| − | :$$X(f)= | + | '''(4)''' Die dazugehörige Spektralfunktion lautet: |
| − | Das Gewicht der Diraclinie bei $f = f_0$ (erster Term) ist $ | + | :$$X(f)={C}/{2}\cdot{\rm e}^{-{\rm j}\varphi}\cdot\delta(f-f_0)+{C}/{2}\cdot{\rm e}^{{\rm j}\varphi}\cdot\delta(f+f_0).$$ |
| + | |||
| + | *Das Gewicht der Diraclinie bei $f = f_0$ (erster Term) ist ${C}/2 \cdot {\rm e}^{–\text{j}\varphi} = 3 \,\text{V} \cdot \cos(60^\circ)- 3 \,\text{V} \cdot \sin(60^\circ)\hspace{0.05cm}\approx \underline{1.5 \,\text{V} - \text{j} \cdot 2.6 \,\text{V}}$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
[[Category:Aufgaben zu Signaldarstellung|^2. Periodische Signale^]] | [[Category:Aufgaben zu Signaldarstellung|^2. Periodische Signale^]] | ||
Aktuelle Version vom 13. April 2021, 15:20 Uhr
Jede harmonische Schwingung kann auch in der Form
- $$x(t)=C\cdot\cos\bigg(2\pi \cdot \frac{t-\tau}{T_0}\bigg)$$
geschrieben werden. Die Schwingung ist somit durch drei Parameter vollständig bestimmt:
- die Amplitude $C$,
- die Periodendauer $T_0$,
- die Verschiebung $\tau$ gegenüber einem Cosinussignal.
Eine zweite Darstellungsform lautet mit der Grundfrequenz $f_0$ und der Phase $\varphi$:
- $$x(t)=C \cdot\cos(2\pi f_0t-\varphi).$$
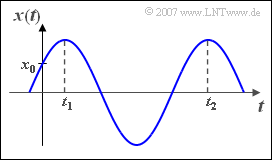
Von einer harmonischen Schwingung ist nun bekannt, dass
- das erste Signalmaximum bei $t_1 = 2 \,\text{ms}$ auftritt,
- das zweite Signalmaximum bei $t_2 = 14 \,\text{ms}$ auftritt,
- der Wert $x_0 ={x(t = 0)} = 3 \,\text{V}$ ist.
Hinweis:
- Die Aufgabe gehört zum Kapitel Harmonische Schwingung.
Fragebogen
Musterlösung
(1) Es gilt $T_0 = t_2 - t_1 = 12\, \text{ms}$ und $f_0 = 1/T_0 \hspace{0.15cm} \underline{\approx 83.33\, \text{Hz}}$.
(2) Die Verschiebung beträgt $\tau \hspace{0.1cm} \underline{= 2\, \text{ms}}$ und die Phase ist $\varphi = 2\pi \cdot \tau/T_0 = \pi/3$ entsprechend $\varphi =\hspace{0.15cm} \underline{60^{\circ}}$.
(3) Aus dem Wert zum Zeitpunkt $t = 0$ folgt für die Amplitude ${C}$:
- $$x_0=x(t=0)=C\cdot\cos(-60\,^\circ)={C}/{2}=\rm 3\,V \hspace{0.3 cm} \Rightarrow \hspace{0.3 cm}\hspace{0.15cm}\underline{\it C=\rm 6\,V}.$$
(4) Die dazugehörige Spektralfunktion lautet:
- $$X(f)={C}/{2}\cdot{\rm e}^{-{\rm j}\varphi}\cdot\delta(f-f_0)+{C}/{2}\cdot{\rm e}^{{\rm j}\varphi}\cdot\delta(f+f_0).$$
- Das Gewicht der Diraclinie bei $f = f_0$ (erster Term) ist ${C}/2 \cdot {\rm e}^{–\text{j}\varphi} = 3 \,\text{V} \cdot \cos(60^\circ)- 3 \,\text{V} \cdot \sin(60^\circ)\hspace{0.05cm}\approx \underline{1.5 \,\text{V} - \text{j} \cdot 2.6 \,\text{V}}$.