Aufgaben:Aufgabe 4.14Z: Auffinden von Echos

• Zurück zum Buch

Messvorrichtung zum Auffinden von Echos

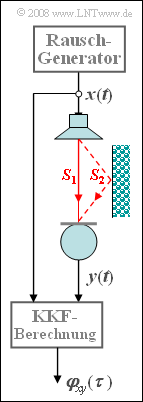
Zur Messung akustischer Echos in Räumen – zum Beispiel bedingt durch Reflexionen an einer Wand – kann die nebenstehende Anordnung verwendet werden.

• Der Rauschgenerator erzeugt ein „im relevanten Frequenzbereich Weißes Rauschen” $x(t)$ mit der Rauschleistungsdichte $N_0 = 10^{-6} \hspace{0.05cm} \rm W/Hz$.
• Dieses ist bandbegrenzt auf $B_x = 20 \hspace{0.05cm} \rm kHz$ und wird auf einen Lautsprecher gegeben.
• Die gesamte Messeinrichtung ist für den Widerstandswert $R = 50 \hspace{0.05cm} \rm \Omega$ ausgelegt.

Das vom Mikrofon aufgenommene Signal ist im allgemeinsten Fall wie folgt beschreibbar:

$$y(t) = \sum_{\mu = 1}^M \alpha_\mu \cdot x ( t - t_\mu ) .$$

Hierbei bezeichnen $\alpha_\mu$ Dämpfungsfaktoren und $t_\mu$ Laufzeiten.

Hinweise:

• Die Aufgabe gehört zum Kapitel Kreuzkorrelationsfunktion_und_Kreuzleistungsdichte.
• Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
• Benutzen Sie für numerische Berechnungen die Parameterwerte

$$\alpha_1 = 0.5, \hspace{0.2cm}t_1 = 200 \,{\rm ms}, \hspace{0.2cm} \alpha_2 = 0.1, \hspace{0.2cm}t_2 = 250 \,{\rm ms}.$$

Fragebogen

1

Geben Sie die AKF $\varphi_x(\tau)$ am Sender an. Wie lautet diese umgerechnet auf den Widerstand $R = 50 \hspace{0.05cm} \rm \Omega$? Wie groß ist der Effektivwert $\sigma_x$?

$\sigma_x \ = $ $\ \rm V$

2

Berechnen Sie die Kreuzkorrelationsfunktion (KKF) $\varphi_{xy}(\tau)$ zwischen Sende– und Empfangssignal. Welche Werte ergeben sich für $\tau = 0$, $\tau = t_1 = 200 \hspace{0.05cm} \rm ms$ und $\tau = t_2$ = 250 \hspace{0.05cm} \rm ms?

$\varphi_{xy}(\tau= 0) \ = $ $\ \rm V^2$

$\varphi_{xy}(\tau= t_1) \ = $ $\ \rm V^2$

$\varphi_{xy}(\tau= t_2) \ = $ $\ \rm V^2$

3

Berechnen Sie das Kreuzleistungsdichtespektrum (KLDS) ${\it \Phi}_{xy}(f)$. Welcher Wert ergibt sich bei der Frequenz $f = 0$?

${\it \Phi}_{xy}(f =0)\ = $ $\ \cdot 10^{-6}\ \rm V^2\hspace{-0.1cm}/Hz$

4

Welche der nachfolgenden Aussagen sind zutreffend, wenn Sie anstelle der in (1) berechneten AKF die Näherung $\varphi_{xy}(\tau) \approx N_0/2 \cdot \delta(\tau)$ verwenden?

	Das Rauschen ist nun „echt” weiß – also nicht bandbegrenzt.
	Die Rauschleistung wird gegenüber der Teilaufgabe (1) vermindert.
	Die Kreuzkorrelationsfunktion ist die Summe gewichteter und verschobener Diracs.
	Das Kreuzleistungsdichtespektrum ist wie in der Teilaufgabe (3) berechnet.

5

Berechnen Sie unter Verwendung der Näherung $\varphi_{xy}(\tau) \approx N_0/2 \cdot \delta(\tau)$ die AKF $\varphi_y(\tau)$. Welche Gewichte ergeben sich für $\tau = 0$ und $\tau = \Delta t = t_2-t_1$?

$\varphi_{y}(\tau= 0) \ = $ $\ \cdot 10^{-6}\ \rm W\hspace{-0.1cm}/Hz$

$\varphi_{y}(\tau= \Delta t) \ = $ $\ \cdot 10^{-6}\ \rm W\hspace{-0.1cm}/Hz$

Musterlösung

Musterlösung

(1)  Das zweiseitige Leistungsdichtespektrum ${\it \Phi}_{x}(f)$ ist im Bereich $\pm B_x$ konstant gleich $N_0/2$. Dessen Fouriertransformierte ergibt die AKF:

$$\varphi_x (\tau) = {N_0}/{2} \cdot 2 B_x \cdot {\rm si} (2 \pi B_x \tau) = 0.02 \hspace {0.05cm}{\rm W} \cdot {\rm si} (2 \pi B_x \tau).$$

Umgerechnet von $R = 50 \hspace{0.05cm} \rm \Omega$ auf $R = 1 \hspace{0.05cm} \rm \Omega$ erhält man somit (Multiplikation mit $R = 50 \hspace{0.05cm} \rm \Omega$):

$$\varphi_x (\tau) = 0.02 \hspace {0.05cm}{\rm VA} \cdot 50 \hspace {0.05cm}{\rm V/A}\cdot {\rm si} (2 \pi B_x \tau)= 1 \hspace {0.05cm}{\rm V}^2 \cdot {\rm si} (2 \pi B_x \tau).$$

Der Effektivwert ist die Wurzel aus dem AKF-Wert bei $\tau = 0$:   $\sigma_x \hspace{0.15cm}\underline{= 1 \hspace {0.05cm}{\rm V}}.$

(2)  Für die Kreuzkorrelationsfunktion (KKF) gilt im vorliegenden Fall:

$$\varphi_{xy} (\tau) = \overline {x(t) \hspace{0.05cm}\cdot \hspace{0.05cm}y(t+\tau)} = \overline {x(t) \hspace{0.05cm}\cdot \hspace{0.05cm}\left [ \alpha_1 \cdot x(t- t_1+ \tau)\hspace{0.1cm}+\hspace{0.1cm} \alpha_2 \cdot x(t- t_2+ \tau)\right] } . $$

Nach Aufspaltung der Mittelwertbildung auf die beiden Terme erhält man hieraus:

$$\varphi_{xy} (\tau) = \alpha_1 \cdot \overline {x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} x(t- t_1+ \tau)} \hspace{0.1cm}+\hspace{0.1cm} \alpha_2 \cdot \overline {x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} x(t- t_2+ \tau)} .$$

Unter Verwendung der AKF $\varphi_x(\tau)$ kann hierfür auch geschrieben werden:

$$\varphi_{xy} (\tau) = \alpha_1 \cdot {\varphi_{x}(\tau- t_1)} \hspace{0.1cm}+\hspace{0.1cm} \alpha_2\cdot {\varphi_{x}(\tau- t_2)} = 1 \hspace {0.05cm}{\rm V}^2 \cdot \left[ \alpha_1 \cdot {\rm si} (2 \pi B_x (\tau - t_1)) + \alpha_2 \cdot {\rm si} (2 \pi B_x (\tau - t_2)) \right].$$

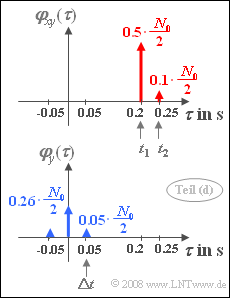
Die si-Funktion weist äquidistante Nulldurchgänge bei allen Vielfachen von $1/(2B_x) = 25 \hspace{0.05cm} \mu s$ auf, jeweils bezogen auf deren Mittellagen bei $t_1 = 200 \hspace{0.05cm} ms$ bzw. $t_2 = 250 \hspace{0.05cm} ms$. Daraus ergeben sich die KKF-Werte zu:

$$\varphi_{xy} (\tau = 0) \hspace{0.15cm}\underline{= 0},\hspace{0.5cm}\varphi_{xy} (\tau = t_1)= \alpha_1 \cdot \varphi_{x} (\tau = 0) \hspace{0.15cm}\underline{= 0.5\,{\rm V}^2} ,\hspace{0.5cm} \varphi_{xy} (\tau = t_2)= \alpha_2 \cdot \varphi_{x} (\tau = 0) \hspace{0.15cm}\underline{= 0.1\,{\rm V}^2} .$$

(3)  Das Kreuzleistungsdichtespektrum (KLDS) ist die Fouriertransformierte der KKF, ebenso wie das Leistungsdichtespektrum (LDS) die Fouriertransformierte der AKF angibt. Für dieses gilt:

$${\it \Phi}_{xy} (f) = \alpha_1 \cdot {\it \Phi}_{x} (f) \cdot {\rm e}^{-{\rm j}2 \pi f t_1} \hspace{0.15cm}+ \hspace{0.15cm}\alpha_2 \cdot {\it \Phi}_{x} (f) \cdot {\rm e}^{-{\rm j}2 \pi f t_2}. $$

Außerhalb des Bereichs $|f| \le B_x$ ist das LDS ${\it \Phi}_{x}(f)$ - und dementsprechend auch das KLDS ${\it \Phi}_{xy}(f)$ - identisch $0$. Innerhalb dieses Intervalls gilt dagegen ${\it \Phi}_{x}(f) = N_0/2$. Daraus folgt in diesem Bereich:

$${\it \Phi}_{xy} (f) = {N_0}/{2} \left( \alpha_1 \cdot {\rm e}^{-{\rm j}2 \pi f t_1} \hspace{0.15cm}+ \hspace{0.15cm}\alpha_2 \cdot {\rm e}^{-{\rm j}2 \pi f t_2} \right). $$

Es ist ersichtlich, dass ${\it \Phi}_{xy}(f)$ im Gegensatz zu ${\it \Phi}_{x}(f)$ eine komplexe Funktion ist. Bei $f = 0$ gilt:

$${\it \Phi}_{xy} (f = 0) = {N_0}/{2} \left( \alpha_1 \hspace{0.15cm}+ \hspace{0.15cm}\alpha_2 \right) = 0.3 \cdot 10^{-6}\hspace{0.05cm}{\rm W/Hz} \hspace{0.15cm}\underline{= 15 \cdot 10^{-6}\hspace{0.07cm}{\rm V^2/Hz}} . $$

(4)  Richtig sind demnach die Lösungsvorschläge 1 und 3:

• Die Fouriertransformierte einer diracförmigen AKF führt zu einem für alle Frequenzen $f$ konstanten LDS, das heißt tatsächlich zu „ echt Weißem Rauschen”. Dieses besitzt eine unendlich große Leistung, und für die KKF kann dann entsprechend der oberen Grafik geschrieben werden:

$$\varphi_{xy} (\tau) = \alpha_1 \cdot { N_0}/{2} \cdot {\rm \delta}( \tau - t_1) \hspace {0.1cm}+ \hspace {0.1cm} \alpha_2 \cdot { N_0}/{2} \cdot {\rm \delta}( \tau - t_2) .$$

• Im Frequenzbereich ist für $|f| \le B_x$ tatsächlich kein Unterschied gegenüber Teilaufgabe (3) feststellbar. Da nun aber echt weißes Rauschen vorliegt, ist aber hier das KLDS nicht auf diesen Bereich beschränkt.

(5)  Für die AKF des echobehafteten Signals gilt:   $\varphi_{y} (\tau) = \overline {y(t) \hspace{0.05cm}\cdot \hspace{0.05cm}y(t+\tau)}$. Diese AKF $\varphi_{y} (\tau)$ lässt sich demzufolge als die folgende Summe darstellen:

$$\alpha_1^2 \cdot \overline {x(t - t_1) \cdot x(t - t_1+ \tau)} \hspace{0.03cm} + \hspace{0.03cm} \alpha_1\hspace{0.02cm}\alpha_2 \cdot \overline {x(t - t_1) \cdot x(t - t_2+ \tau)} + \hspace{0.05cm} \alpha_2\hspace{0.02cm}\alpha_1 \cdot \overline {x(t - t_2) \cdot x(t - t_1+ \tau)}\hspace{0.03cm} + \hspace{0.03cm} \alpha_2^2 \cdot \overline {x(t - t_2) \cdot x(t - t_2+ \tau)}. $$

Für den ersten und den letzten Mittelwert gilt:

$$\overline {x(t - t_1) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t - t_1+ \tau)} = \overline {x(t - t_2) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t - t_2+ \tau)} = \overline {x(t ) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t + \tau)} =\varphi_x(\tau).$$

Dagegen erhält man für den zweiten und den dritten Mittelwert mit $\Delta t = t_2 - t_1= 50 \, \rm ms$:

$$\overline {x(t - t_1) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t - t_2+ \tau)} = \overline {x(t ) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t + t_1- t_2+ \tau)} =\varphi_x(\tau - \Delta t),$$

$$\overline {x(t - t_2) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t - t_1+ \tau)} = \overline {x(t ) \hspace{0.05cm}\cdot \hspace{0.05cm}x(t + t_2- t_1+ \tau)} =\varphi_x(\tau + \Delta t).$$

Insgesamt ergibt sich somit wieder eine symmetrische AKF, wie in der unteren Grafik dargestellt:

$$\varphi_{y} (\tau) = {N_0}/{2} \cdot \left[ ( \alpha_1^2 \hspace{0.1cm} + \hspace{0.1cm} \alpha_2^2 ) \cdot {\rm \delta} (\tau) \hspace{0.1cm} + \hspace{0.1cm} \alpha_1 \cdot \alpha_2 \cdot {\rm \delta}(\tau - \Delta t) \hspace{0.1cm} + \hspace{0.1cm} \alpha_1 \cdot \alpha_2 \cdot {\rm \delta}(\tau + \Delta t) \right].$$

$$\Rightarrow \hspace{0.3cm}\varphi_{y} (\tau = 0 ) \hspace{0.15cm}\underline{= 0.13 \cdot 10^{-6}\, {\rm W/Hz}}, \hspace{0.3cm}\varphi_{y} (\tau = \Delta t )\hspace{0.15cm}\underline{ = 0.025 \cdot 10^{-6}\, {\rm W/Hz}}.$$