Aufgaben:Aufgabe 2.4: 2D-Übertragungsfunktion

• Zurück zum Buch

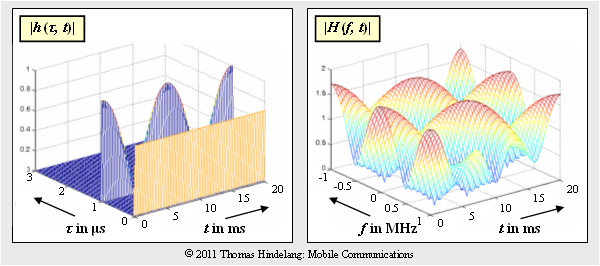
Dargestellt ist die zweidimensionale Impulsantwort $h(\tau, t)$ eines Mobilfunksystems in Betragsdarstellung. Es ist zu erkennen, dass die 2D–Impulsantwort nur für die Verzögerungszeiten $\tau = 0$ und $\tau = 1 \ \rm \mu s$ Anteile besitzt. Zu diesen Zeitpunkten gilt:

$$h(\tau = 0\,{\rm \mu s},\hspace{0.05cm}t) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} = {\rm const.}$$

$$h(\tau = 1\,{\rm \mu s},\hspace{0.05cm}t) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \cos(2\pi \cdot {t}/{ T_0})\hspace{0.05cm}.$$

Für alle anderen $\tau$–Werte ist $h(\tau, t) = 0$.

Gesucht ist die zweidimensionale Übertragungsfunktion $H(f, t)$ als die Fouriertransformierte von $h(\tau, t)$ hinsichtlich der Verzögerungszeit $\tau$:

$$H(f,\hspace{0.05cm} t) \hspace{0.2cm} \stackrel {f,\hspace{0.05cm}\tau}{\bullet\!\!-\!\!\!-\!\!\!-\!\!\circ} \hspace{0.2cm} h(\tau,\hspace{0.05cm}t) \hspace{0.05cm}.$$

Hinweise:

• Die Aufgabe gehört zum Kapitel Mehrwegeempfang beim Mobilfunk.
• Eine ähnliche Problematik wird in der Aufgabe A2.5 behandelt, allerdings mit veränderter Nomenklatur.

Fragebogen

1

Wie groß ist die Periodendauer $T_0$ der Funktion $h(\tau = 1 \ {\rm \mu s}, t)$? Beachten Sie, dass in der Grafik der Betrag $|h(\tau, t)|$ dargestellt ist.

$T_0 \ = \ $ $\ \rm ms$

2

Zu welchen Zeiten $t_1$ (zwischen $0$ und $10 \ \rm ms$) und $t_2$ (zwischen $10 \ \rm ms$ und $20 \ \rm ms$) ist $H(f, t)$ bezüglich $f$ konstant?

$t_1 \ = \ $ $\ \rm ms$

$t_2 \ = \ $ $\ \rm ms$

3

Berechnen Sie $H_0(f) = H(f, t = 0)$. Welche Aussagen sind zutreffend?

	Es gilt $H_0(f) = H_0(f + i \cdot 1 \ {\rm MHz}), i = ±1, ±2, \ ...$
	Es gilt näherungsweise $0.293 ≤ |H_0(f)| ≤ 1.707$.
	$|H_0(f)|$ hat bei $f = 0$ ein Maximum.

4

Berechnen Sie $H_{10}(f) = H(f, t = 10 \ \rm ms)$. Welche Aussagen sind zutreffend?

	Es gilt $H_{10}(f) = H_{10}(f + i \cdot 1 \ \rm MHz), i = ±1, ±2, \ ...$
	Es gilt näherungsweise $0.293 ≤ H_{10}(f) ≤ 1.707$.
	$|H_{10}(f)|$ hat bei $f = 0$ ein Maximum.

Musterlösung

Musterlösung

(1)  Die Periodendauer kann man aus der gegebenen Gleichung bestimmen. Berücksichtigt man die Betragsdarstellung, so ergibt sich $T_0 \ \underline {= 20 \ \rm ms}$.

(2)  Zum Zeitpunkt $t_1 \ \underline {= 5 \ \rm ms}$ ist $h(\tau = 1 \ {\rm \mu s}, t_1) = 0$. Dementsprechend gilt

$$h(\tau = 1\,{\rm \mu s},\hspace{0.05cm}t_1) = \frac{1}{ \sqrt{2}} \cdot \delta(\tau)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} H(f,\hspace{0.05cm}t_1) = \frac{1}{ \sqrt{2}} = {\rm const.}$$

Ebenso gilt für $t_2 \ \underline {15 ms}$:

$$h(\tau = 1\,{\rm \mu s},\hspace{0.05cm}t_2) = \frac{1}{ \sqrt{2}} \cdot \delta(\tau)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} H(f,\hspace{0.05cm}t_2) = \frac{1}{ \sqrt{2}} = {\rm const.}$$

(3)  Zum Zeitpunkt $t = 0$ lautet die Impulsantwort mit $\tau_1 = 1 \ \rm \mu s$:

$$h(\tau,\hspace{0.05cm}t = 0) = \frac{1}{ \sqrt{2}} \cdot \delta(\tau)+ \delta(\tau - \tau_1)\hspace{0.05cm}.$$

Die Fouriertransformation führt zum Ergebnis:

$$H_0(f) = H(f,\hspace{0.05cm}t = 0) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} + 1 \cdot {\rm exp}(- {\rm j}\cdot 2 \pi f \tau_1)=$$

$$\hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} + \cos( 2 \pi f \tau_1)- {\rm j}\cdot \sin( 2 \pi f \tau_1)$$

$$\Rightarrow \hspace{0.3cm} |H_0(f)| \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sqrt { \left [ {1}/{ \sqrt{2}} + \cos( 2 \pi f \tau_1) \right ]^2 + \left [\sin( 2 \pi f \tau_1)\right ]^2}=$$

$$\hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sqrt { 0.5 + 1 + {2}/{ \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)} = \sqrt { 1.5 + { \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)}\hspace{0.05cm}.$$

Daraus folgt:

• $H_0(f)$ ist periodisch mit $1/\tau_1 = 1 \ \rm MHz$.
• Für den Maximalwert bzw. Minimalwert gilt:

$${\rm Max}\, \left [ \, |H_0(f)|\, \right ] \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sqrt { 1.5 + { \sqrt{2}} } \approx 1.707 \hspace{0.05cm},$$

$${\rm Min}\, \left [ \, |H_0(f)|\, \right ] \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sqrt { 1.5 - { \sqrt{2}} } \approx 0.293 \hspace{0.05cm}. $$

• Bei $f = 0$ hat $|H_0(f)$ ein Maximum.

Richtig sind demzufolge alle drei Lösungsvorschläge.

(4)  Für den Zeitpunkt $t = 10 \ \rm ms$ gelten folgende Gleichungen:

$$h(\tau,\hspace{0.05cm}t = 10\,{\rm ms}) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} \cdot \delta(\tau)- \delta(\tau - \tau_1)\hspace{0.05cm},$$

$$H_{10}(f) = H(f,\hspace{0.05cm}t = 10\,{\rm ms}) \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \frac{1}{ \sqrt{2}} - \cos( 2 \pi f \tau_1)+ {\rm j}\cdot \sin( 2 \pi f \tau_1)\hspace{0.05cm},$$

$$ |H_{10}(f)| \hspace{-0.1cm} \ = \ \hspace{-0.1cm} \sqrt { 1.5 - { \sqrt{2}} \cdot \cos( 2 \pi f \tau_1)}\hspace{0.05cm}.$$

Die Frequenzperiode ändert sich gegenüber $t = 0$ nicht. Der Maximalwert ist weiterhin $1.707$ und auch der Minimalwer $0.293$ ändert sich nicht gegenüber der Teilaufgabe (3). Bei $f = 0$ tritt nun allerdings ein Minimum und kein Maximum auf ⇒ Richtig sind die Lösungsvorschläge 1 und 2.

Die rechte Grafik zeigt den Betrag $|H(f, t)|$ der zweidimensionalen Übertragungsfunktion.